The D2h Point Group
his point group contains the following symmetry operations:
E the identity operation
C2 a twofold principal symmetry axis
2 * C2 two twofold symmetry axes orthogonal to the principal axis
i inversion through a center of symmetry
σh a horizontal mirror plane intersecting the principal symmetry axis
2 * σv two vertical mirror planes aligned with the principal symmetry axis
A simple example for a D2h symmetric molecule is ethylene (C2H4), here in its HF/6-31G(d) optimized structure:
#P RHF/6-31G(d) opt=(Z-Matrix,tight)
RHF/6-31G(d) opt min ethylene D2h sym.
0 1
X1
X2 1 1.0
C3 2 r3 1 90.0
C4 2 r3 1 90.0 3 180.0
H5 3 r5 2 a5 1 -90.0
H6 3 r5 2 a5 1 90.0
H7 4 r5 2 a5 1 -90.0
H8 4 r5 2 a5 1 90.0
r3=0.65846705
r5=1.07598871
a5=121.81387521
|
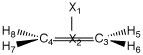 |
In this case the symmetry of the system is reflected in the Z-Matrix through the use of identical variable names for hydrogen atoms H5 - H8 and through constraining all atoms to the symmetry plane. This reduces the number of independent structural variables from 12 (for an asymmetric, non-linear molecule containing six centers) to 3 and thus accelerates geometry optimizations.
Molecular orbitals as well as harmonic vibrations (if calculated) are labeled according to their symmetry properties as belonging to one of the eight irreducible representations (Ag, Au, B1g, B2g, B3g, B1u, B2u, B3u) of the D2h point group.